Robustness of quantum mechanics under perturbations investigated
6 February 2017

Dicke model
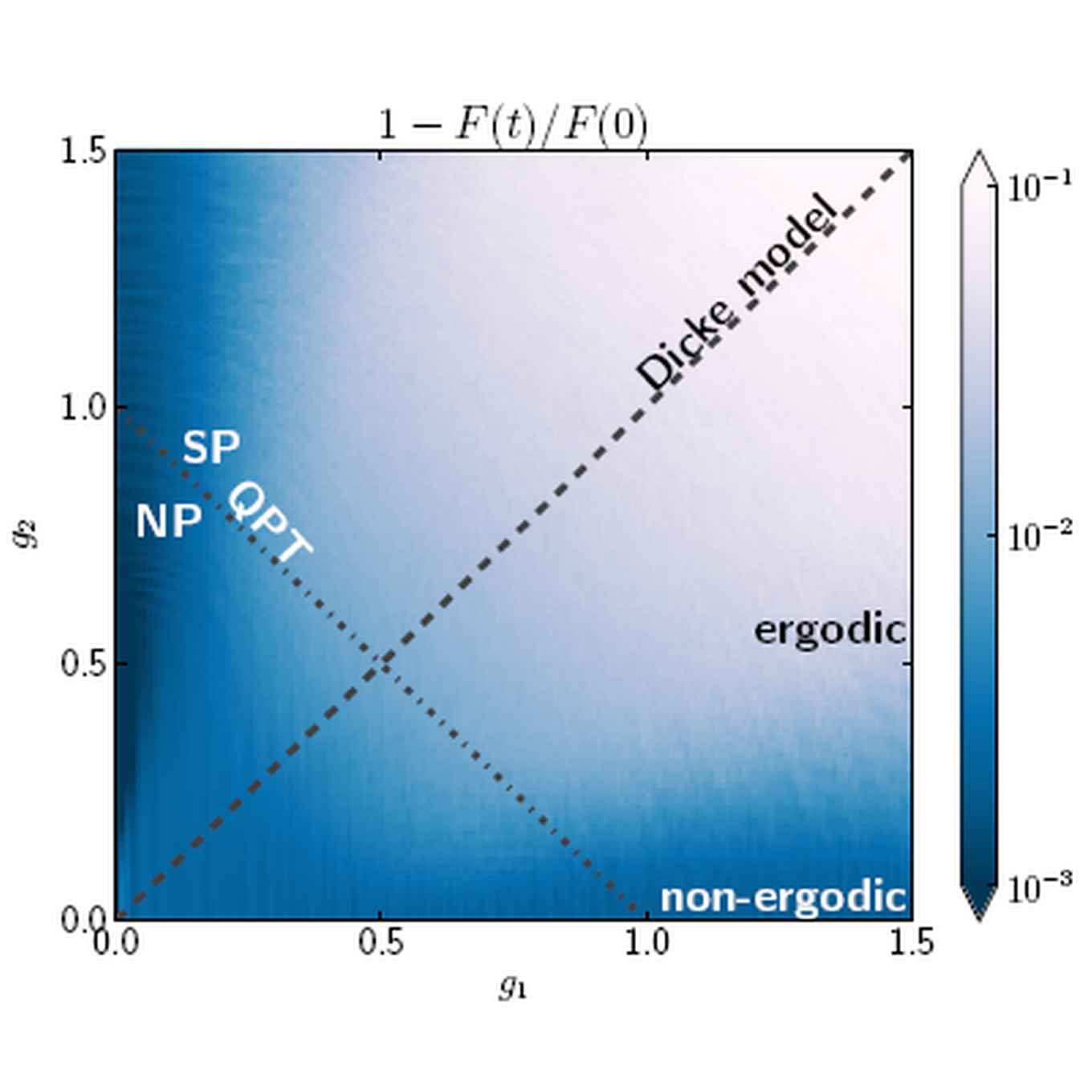
In contrast to the domain of classical mechanics, in quantum mechanics there is no simple answer to the fundamental question to what extent the dynamical properties of a system change under a perturbation. In the article 'Nonergodicity in the Anisotropic Dicke Model', to appear in Physical Review Letters this week, new evidence for the existence of a quantum mechanical equivalent of the KAM theorem is presented. In their article, the authors study the robustness of a specific quantum mechanical model, the so called Dicke model, under perturbations. This model is often used to describe interactions between light and matter, but also to investigate the connections between classical and quantum chaotic behavior.
Quantum KAM theorem
The research on the Dicke model is based on the Master's thesis of Wouter Buijsman. Buijsman, who is now a PhD student in the Delta Institute for Theoretical Physics, carried out the research with his supervisors Vladimir Gritsev and Rudolf Sprik. The researchers show that, as in classical mechanics, the system only shows quantum chaotic behaviour under sufficiently large perturbations. This observation constitutes a new clue towards the existence of a quantum mechanical equivalent of the KAM theorem. The researchers are very pleased with the results, and hope it will be followed up in future investigations.
Reference
Non-ergodicity in the Anisotropic Dicke model, W. Buijsman, V. Gritsev and R. Sprik. To appear in Physics Review Letters this week.
Update: Phys. Rev. Lett. 118, 080601, 23 February 2017, https://doi.org/10.1103/PhysRevLett.118.080601.